Written by: Nils Heinonen, Argonne Leadership Computing Facility
Recent advances in quantum Monte Carlo (QMC) methods have the potential to revolutionize computational materials science, a discipline traditionally driven by density functional theory (DFT). While DFT—an approach that uses quantum-mechanical modeling to examine the electronic structure of complex systems—provides convenience to its practitioners and has unquestionably yielded a great many successes throughout the decades since its formulation, it is not without shortcomings, which have placed a ceiling on the possibilities of materials discovery. QMC is poised to break this ceiling.
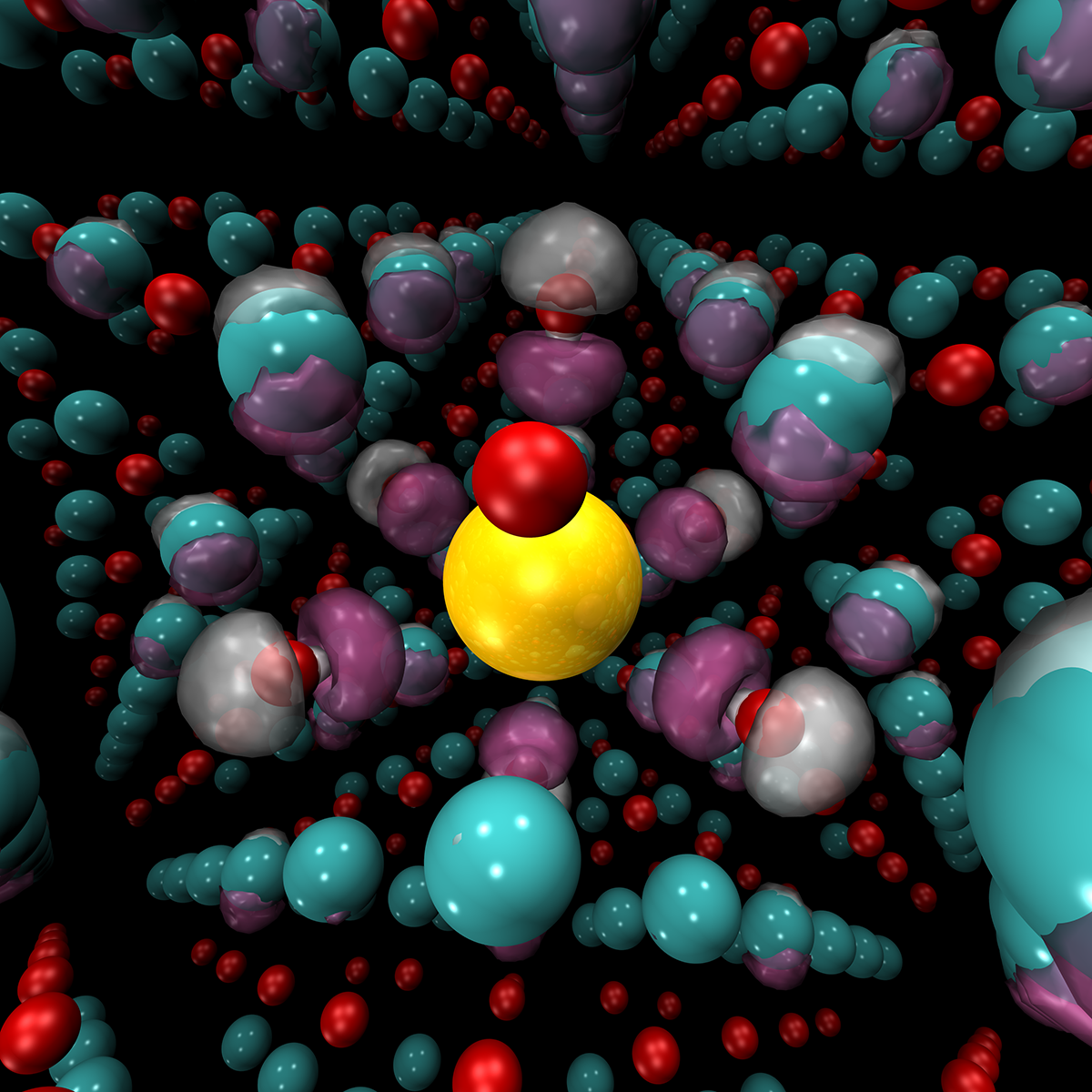
The key challenge is to solve the quantum many-body problem accurately and reliably enough for a given material. QMC solves these problems via stochastic sampling—that is, by using random numbers to sample all possible solutions. The use of stochastic methods allows the full many-body problem to be treated while circumventing large approximations. Compared to traditional methods, they offer extraordinary potential accuracy, strong suitability for high-performance computing, and—with few known sources of systematic error—transparency. For example, QMC satisfies a mathematical principle that allows it to set a bound for a given system’s ground state energy (the lowest-energy, most stable state).
QMC’s accurate treatment of quantum mechanics is very computationally demanding, necessitating the use of leadership-class computational resources and thus limiting its application. Access to the computing systems at the Argonne Leadership Computing Facility (ALCF) and the Oak Ridge Leadership Computing Facility (OLCF)—U.S. Department of Energy (DOE) Office of Science User Facilities—has enabled a team of researchers led by Paul Kent of Oak Ridge National Laboratory (ORNL) to meet the steep demands posed by QMC. Supported by DOE’s Innovative and Novel Computational Impact on Theory and Experiment (INCITE) program, the team’s goal is to simulate promising materials that elude DFT’s investigative and predictive powers.
+ Read the full feature: https://www.alcf.anl.gov/articles/predicting-material-properties-quantum-monte-carlo